Last year’s experience of retaking the SATs reminded me of a mathematical mystery left over from high school, namely why dividing a number by zero is either not possible or equals infinity. I never understood the logic behind this, but begrudgingly accepted it as a rule I had to live by. My day job of medical consulting is currently in a bit of a lull, so I decided to use this found time to spend quality time with zero and grok it.
In high school I assumed that if I didn’t understand something it was my fault, but as I investigated zero I was astonished to find that zero has perplexed mathematicians, philosophers and religious leaders for thousands of years. Part of the reason was that there was no role for zero in early math,
which was simply based on counting – Ooga Magook in his cave counting bears’ skins, or Jesus counting his shepherds, and his shepherds counting their sheep. If there were no bearskins, there was simply nothing to count, and Mr. Magook would merely grunt, “I got no skins.”
My high school thinking was not much more sophisticated than the Neanderthals– I was a counter and to me zero meant nothing, and therefore dividing by zero should be the same thing as not dividing at all. Six divided by zero should remain six. On the other hand multiplying by zero made sense – 6 x 0 is the same thing as adding six zeros, and I don’t care how many zeros you add, you are still going to come up with nothing. Another way to appreciate the dilemma is to recall that division should reverse multiplication. For example, if you divide 6 by 2 (i.e. = 3) and then multiply the results by 2 (i.e. 3×2), you get back to your original 6. If you put the two steps into the same equation we get:
6 x 2/2
which of course we immediately recognize as 6×1=6.
But look what happens when we apply the same logic to dividing by zero. If we divide 6 by 0 and then multiply by 0 we should get back to our original 6. But wait – we know that multiplying anything by zero equals zero, so we can never get back to our original 6. Our combined equation is 6 x 0/0= 0, which makes sense I suppose because 0/0 should not equal 1.
But this doesn’t tell us what 6 divided by 0 actually is, all we really know is that multiplying any number by zero becomes zero. I have certainly progressed beyond Ooga counting his bear skins, but I am still not grokking it. I want to know the solution to 6 divided by zero – how can it be infinity?
The above brainteaser treats zero as a something beyond a pure counting number, i.e. zero is both a number and a concept. However, early numbering systems did not even include a zero as a counting number. I naively thought the Roman numerals, which of course include no zero, were only used to decorate the base of buildings. After all, how could the Romans possibly add, subtract, multiply or divide with this clumsy notation? It turns out that this was indeed a serious problem for toga’ed merchants who had to resort to using their fingers or perhaps an abacus to conduct business. The result was that there could be no written record of transactions, and if you didn’t believe Caesar’s calculation, you had no recourse. There was no such thing as an accountant.
We blithely take for granted our current the place-value number system (i.e. a slot for the ones, tens, hundreds, etc) that was developed in India around 600 AD, and brought west by the Arabs as they traded along the Silk Road. But in this notation, zero was merely a placeholder and was not regarded as a number with properties. Among the Greeks and Romans, resistance to the concept of zero was based on the troublesome implications of zero symbolizing nothingness or a void. Equally troublesome was its twin, infinity, symbolizing an endless universe. And the acceptance of both undermined the concept of an omnipotent God. Aristotle was the most prominent Greek philosopher and he adamantly rejected both zero and infinity. He envisioned the universe as a finite
number of orbs spinning around a centrally located earth. Since there was no infinity, there had to be an outer limit of the orbs, and this consisted of a sphere studding with glowing lights, i.e. the stars. Since there was no such thing as a void, someone had to set the orbs spinning, and that of course an God putting everything in motion. Aristotle’s concepts became closely allied with Christianity, and became the dominant philosophy in the Western world for over 2,000 years until the Reformation in the 16th century.
Unencumbered by Aristotelian philosophy, I think that we can all agree on some sort of concept of zero and infinity. But Artistotle’s universe has left an interesting heritage, and our calendars are the most glaring example. Calendars are basically timelines and thus need a starting point, which for Western civilization is the birth of Christ. This was assigned the date 1 AD, and the first year before the birth of Christ was 1 BC. Sounds logical, but except for the tiny detail of zero. There was no zero year. If you put the calendar on a number line, the numbers would go directly from 1 to -1, instead of 1, 0, -1 on the number line illustrated below.
Now, if you are asked how years are there between 10 BC and 10 AD, the most logical answer would be 20, but in reality, there are only 19 years since the year 0 is skipped. One testament to grokking is the ability to confidently explain a problem in multiple different ways, so here’s another approach. Designating 1 AD as the year that Jesus born would be similar to saying that at the moment of your birth you are 1 years old. This is of course stupid, because to determine age, our calendar starts at zero – we only turn 1 when we have completed our yearly lap around the sun.
The lack of a year zero in calendars is the reason why our centuries do not synch up with the years. For example, we are currently in the 21stcentury, but the years in this century range from 2000 to 2100. This little fluke also leads to the discussion of when a century ends. Should we have celebrated on December 31, 1999 or December 31, 2000? Technically we should have celebrated in 2000. Other little leftovers from the lack of a zero includes the way we teach kids to count, , i.e. 1,2,3,4…. , when it should really be 0,1,2,3,4. Telephones typically position the “0” key at the base of the pad, following 9, and as I look at my keyboard I realize that the “0” is at the far right. Technically both wrong.
I now feel totally grokked on the history of zero, and the surprising role of Christianity in stifling its acceptance. This brings me up to about 1665 when Isaac Newton flings open the door to both zero and infinity and ushers in a period of phenomenal growth in math sophistication. It is called
Calculus, and one of its breakthroughs was how to set limits on infinite numbers. For example, in one of Newton’s basic calculations (determining the slope of a tangent to a circle) he had to decide how to manage the square of an infinitesimal fraction, which of course is an even smaller than infinitesimal. He basically ignored it and treated this teensie number as if it was zero – this fudge factor was the basis of calculus. The same strategy can be applied to dividing a number by zero. According to Calculus you should consider what happens as the denominator (the bottom number) approaches zero and gets smaller and smaller. The basic principle is that the nominator (top number) gets larger as the denominator gets smaller.
1 ÷ 2 = 1/2 but
1 ÷ 1/2 = 2, and
1 ÷ 1/4 = 4, and
1 ÷ 1/100 = 100, and so on ad infinitum
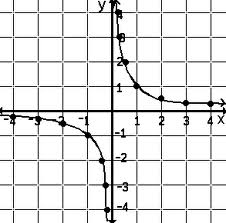
So as you divide by infinitesimally smaller fractions, the numerator accordingly approaches infinity. At first this seemed to solve my dilemma – I could feel comfortable saying that dividing anything by zero is infinity. In fact, we probably don’t need to know what will exactly happen when we reach zero, because we will be so infinitesimally close. (See the upper right quadrant of the below graph – the value of Y soars upward towards infinity as you approach zero on the X axis) After stumbling, fumbling and rumbling through the history of math, it seems easy – I have grokked it – dividing by zero equals infinity.
But my triumph immediately slipped through my grasp as I read on in my reference book. Look what happens if you approach zero from the negative (-1) side of things, i.e. divide 1 by smaller and smaller negative fractions as you approach zero from the left. (See lower left hand quadrant in the above graph) You will great increasingly larger negativenumbers that will approach negative infinity! Negative infinity – what the hell is that?? How can dividing by zero essentially result in two different diametrically opposed numbers – negative and positive infinity?? It makes me want to scurry back to the coziness of Ooga Magook’s cave and contentedly count skins with my fingers. But I say to myself, “Suck it up Brown, you just must come to grips that dividing by zero is indefinable.” As we get closer and closer to dividing by zero, we veer off to infinity in one direction or another. We may never know what happens when negative and positive infinity collide at exactly point zero – we could be sucked into a black hole, or be flung out into a separate universe, experience life before birth, or life after death. Grokking the indefinable means that we must be willing to be surprised.
The missing words in the following poem are anagrams (i.e. share the same letters like stop, post, spot) and the number of asterisks indicates that number of letters. One of the missing words will rhyme with either the previous or following lines. Your job is to solve for the missing words based on the above rules and context of the poem. Scroll down for answers.
In Medieval times mathematicians quickly learned that
They must steer clear of the man in the pointed *** ***.
That was a bishop wearing his miter and he was consumed
With ****** for those who beliewed in zero and all it presumed.
The church was desperate to maintain Artistotle’s vision that hung by a *****,
And so in the Counter-Reformation nay-sayers ended up dead.
By the 1600s, only a ***** of people didn’t think that the clergy was nuts,
And finally the church accepted zero and infinity, no ifs ands or buts
*
*
*
*
*
*
*
Answers: Red hat, hatred, thread, dearth
Share:
